lattice polygons | science game

The multicolored illustration of ostomy It would have been possible to solve last week with only three colors without two adjacent pieces of the same color, as evidenced by the figure sent by Salva Foster, which also indicates the cutting areas.
This is the most abbreviated way of expressing the relative areas of the segments with integers, the smallest of which is taken as a unit. If we also want to express the sides in integers, which makes the calculations easier, we can use the twelfth part of the side as a linear unit, so that the area becomes 144 square units (how many of them correspond to the top piece?).
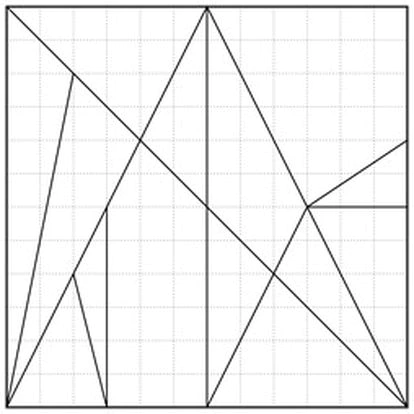
In addition, choosing this unit makes it easier to form shapes, since many sides of the pieces measure an integer number of units, which makes it easy to compare them with each other. Among the shapes that can be built with all the pieces ostomy, a particularly interesting rectangle (in fact, the puzzle sometimes comes in a rectangular box), which, as a hint, I will say is a vertical “break line” that divides it into two equal parts that look like squares (could they be?). Can other rectangles of different dimensions be built? Unlike one, yes, like a 6×24, Fuster (which in vain doesn’t mean a carpenter) also found it using ostomy local. And without investing any parts?

Coincidentally (or maybe not) in this case there is also a central fraction line that divides the rectangle into two equal parts: two 6 x 12 dominoes. Will it always be this way in all possible rectangles (including the square) or can it be built One without vertical break lines?
Beck’s theory
by installing ostomy In a 12×12 grid, its segments become lattice polygons, which are those simple (i.e. without holes) polygons whose vertices coincide with many other vertices of the lattice, which is equivalent to saying that they have integer coordinates with respect to some Cartesian axes.
In 1899, the Austrian mathematician Georg-Alexander Beck proved the theorem that bears his name, making it easy to find the area of a lattice polygon from the number of vertices of the lattice lying within the polygon (i) and the number from the vertices on its sides (p). Thus, in the attached figure i = 15 and p = 10.
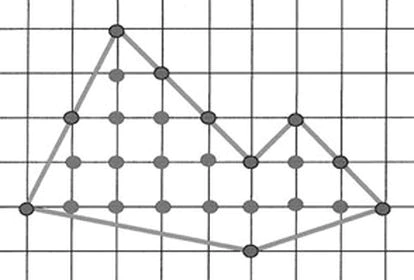
I invite experienced readers to find the simple formula that gives the area of a polygon as a function of i and p. I’m not asking you to prove Beck’s theorem (although it wouldn’t hurt to try), but looking at parts ostomy, in addition to this last figure, find an equation (I insist, very simple) that allows you to find their areas based on the number of their inner points and their perimeter. It is easy to find this network formula in the large network of the Internet; But it’s not about that, but to infer that it’s “Fermi” (as we’ve seen on other occasions, Fermi had a special ability to derive correct conclusions from hashed data). Hint: Pick’s formula is formally similar to Euler’s formula for a polyhedron: C + V = A + 2 (faces plus vertices equals sides plus two).
Speaking of polyhedra, it is tempting to try to extend Beck’s theorem into three dimensions to find the volume of a lattice polyhedron as a function of the number of its interior and boundary points; But in 1957, John Reeve showed that wasn’t possible, and he did so by way of an ingenious counterexample called, in his honor, the Reeve tetrahedron. But this is another article.
Carlo Frappetti Writer, mathematician, and member of the New York Academy of Sciences. He published more than 50 popular scientific works for adults, children and youth, among them “Maldita physics”, “Malditas Matematicas” or “El Gran Juego”. He was the screenwriter of The Crystal Ball.
You can follow Thing On FacebookAnd the Twitter And the Instagramor sign up here to receive Our weekly newsletter




